In our earlier write-up about bond valuation, we addressed how the concept of time value of money plays a big role in figuring out what an asset is truly worth before you decide to invest. Now, let’s take it a step further. I really recommend giving the first part a read if you haven’t already, because it sets the stage for what we’re about to explore next. In this second part of our discussion on bond valuation, we’re going to explore the nitty-gritty details: How exactly do you go about valuing a bond? Let’s jump right in!
Let’s review what we covered earlier. When we talk about valuing a financial asset, it’s all about figuring out how much those future cash flows are worth today. The process can be simplified into three easy steps:
1. Estimate the expected cash flows
2. Determine the appropriate rate of return to discount the cash flows
3. Calculate the present value of the expected cash flows using the rate of return determined. When we input this into a formula, it translates to:

This is how much a future cash flow is worth today.
Now, assuming there are multiple cash flows over a period of time, then the present value will be sum of all expected cash flows.
Present Value of Cash Flow1 + Present Value of Cash Flow2 +….+ Present Value of Cash FlowN
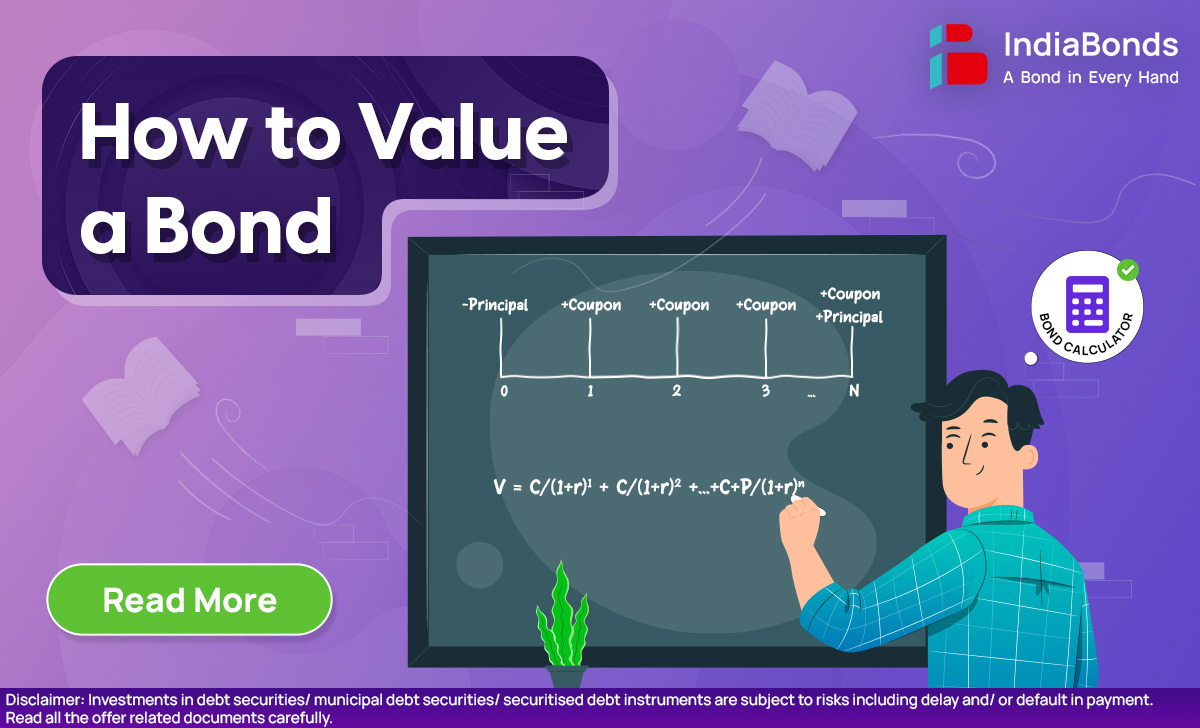
When you invest in a bond, you receive two main types of cash flows: the coupon payment and the principal amount commonly referred to as the par value. These cash flows can be illustrated as follows:

To understand the concept of the present value formula concerning bonds, let’s look at a bond valuation formula with example. Imagine a bond with a maturity period of 4 years, offering a 10% coupon rate and having a par value of Rs 100 at maturity. For simplicity, let’s assume this bond pays coupons annually. Now, to calculate the present value of each cash flow, we apply a discount rate of 8%. The cash flow of this bond will be:
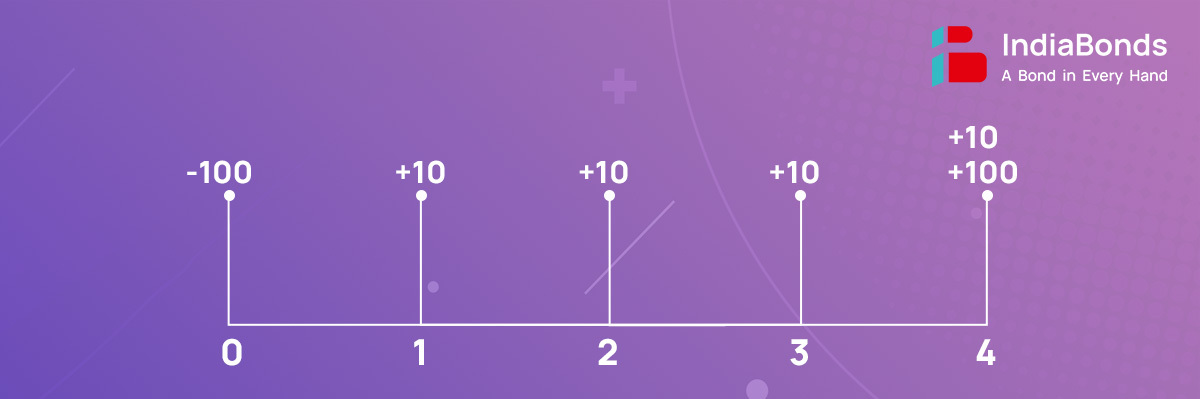
Now, the year 1 discounted cash flow would be 10/(1+8%)1 = Rs 9.2592
This means Rs 10 to be received after 1 year from now would be valued at Rs 9.2592 today (at t = 0) with 8% discount rate.
Similarly, the present value of each cash flow would be:
Year 1: Present Value = 10/(1+.08)1 = Rs. 9.2592
Year 2: Present Value = 10/(1+.08)2 = Rs. 8.5734
Year 3: Present Value = 10/(1+.08)3 = Rs. 7.9383
Year 4: Present Value = 110/(1+.08)4 = Rs. 80.8533
The value of the bond today is the sum of the present values of the four cash flows;
Rs. 9.2592 + Rs. 8.5734 + Rs. 7.9383 + Rs. 80.8533
Rs. 106.6242
Now, suppose the discount rate is increased to 11%. By repeating the steps above, we find that the sum of the present value of discounted cash flows is Rs. 96.8976. But what if the discount rate is at 10%, i.e., the same as the coupon rate? Surprisingly, the sum of the present value of the discounted cash flow is, surprise surprise, Rs. 100, matching the par value. Why does this happen?
From our above bond valuation example, we can state the following:
If Coupon Rate > Discount Rate, Bond Price > Par Value (Premium)
If Coupon Rate < Discount Rate, Bond Price < Par Value (Discount)
If Coupon Rate = Discount Rate, Bond Price = Par Value
So, picture this: You’ve got a bond that’s trading at a premium, meaning it’s priced above its par value. This scenario indicates that its coupon rate is giving out more returns compared to what the market requires. In simpler terms, if you own such a bond with a coupon rate higher than what the market demands, chances are, you can sell it for a higher price.
Conversely, if I’m holding a bond with a coupon rate lower than what the market requires, I might have to sell it at a discount to attract buyers. And you know what? This situation rings a bell—it’s none other than the classic tale of the inverse relationship between bond yield and price.




It’s crucial to avoid confusing the required rate of return with yields, as this is where investors often find themselves in a muddle. Although both yield and the discount rate are expressed as percentages, they serve different purposes in bond valuation.
Yield measures the return generated by holding a bond, taking into account coupon payments and the bond’s market price.
Discount rate is used to determine the present value of a bond’s future cash flows, considering the time value of money and the risk associated with the investment.
The inverse relationship between yields and bond prices is indeed closely related to the concept of present value and discounted cash flows.
Bond yields also known as current yield or yield to market, refers to the yield of a bond based on its current market price
When bond yields rise:
As yields increase, the discount rate used to calculate the present value of a bond’s future cash flows also increases. This means that future cash flows are discounted at a higher rate, resulting in a lower present value for those cash flows. Therefore the current market price of the bond must adjust downward to maintain equilibrium. Investors are willing to pay less for a bond with lower present value cash flows, causing bond prices to fall.
Conversely, when bond yields fall:
The discount rate used to calculate the present value of a bond’s future cash flows decreases. This results in a higher present value for those cash flows and given the higher present value of future cash flows, investors are willing to pay more for the bond, driving its market price higher.
In a nutshell, it’s like a seesaw game between bond yields and prices: when one goes up, the other goes down and vice versa. This establishes an inverse relationship between bond yields and prices.
If you’ve made it this far, congratulations! However, this was just a simple example of bond valuation calculation involving a plain vanilla bond. There are various scenarios involving different types of bonds. For instance, valuing zero coupon bonds or bonds with multiple discount rates, situations where cash flows occur semi-annually, determining the value of a bond between coupon payments and even pricing a bond with default risk. Calculating all of these scenarios on Excel can be quite challenging.
To simplify your life, IndiaBonds is proud to present its very own bespoke Bond Calculator. The IndiaBonds Bond Calculator can perform all of these functions and more when it comes to valuing a bond. Specifically designed for the Indian bond market, it is the first of its kind. It offers features such as built-in cash flows, bond comparison, precise calculations, detailed breakdown of bond components, 24×7 availability, user-friendly interface and many more. It caters to both beginner and experienced bond investors alike. Simply log on to IndiaBonds, sign up and begin your bond investment journey with the bond calculator.
Understanding the present value of discounted cash flows for a bond is like having a superpower for investors. It helps us figure out whether a bond is trading at a premium or a discount compared to what it’s really worth, based on what the market expects in returns and let’s face it, whether we’re investing in bonds, stocks or anything else, knowing how to properly value our investments is key.
Think of valuation as a secret weapon in the investor’s toolkit. The more we master it, the better equipped we are to make smart investment decisions. As Philip Fisher, the renowned investment guru, once said, “The stock market is filled with individuals who know the price of everything, but the value of nothing.” So, let’s not just focus on the price tags; let’s dig deeper to understand the true value of our investments.
A. Bond valuation is the process of determining the fair value of a bond. It involves assessing factors such as coupon rate, market interest rates, maturity date, credit quality, call provisions and market sentiment. This valuation helps investors evaluate whether a bond is overvalued, undervalued or fairly priced in the market, guiding their investment decisions.
A. The bond valuation formula calculates the present value of the bond’s future cash flows, which include periodic coupon payments and the bond’s face value at maturity. The formula is:
V = C/(1+r)1 + C/(1+r)2 +…+C+FV/(1+r)n
Where:
V = Present value of the bond
C = Coupon payment per period
r = Required rate of return
n = Number of periods until maturity
FV = Face value of the bond
This formula discounts each cash flow back to its present value using the discount rate. The sum of all discounted cash flows gives the bond’s fair value.
A. These methods comprise Discounted Cash Flow (DCF), the Yield to Maturity (YTM) method, Credit Spread Analysis, Bond Benchmarking, Option-Adjusted Spread (OAS) Method amongst others. Each method has its advantages and limitations and the choice of valuation method depends on factors such as data availability, bond complexity and investor preferences.
Disclaimer: Investments in debt securities/ municipal debt securities/ securitised debt instruments are subject to risks including delay and/ or default in payment. Read all the offer related documents carefully.